A common way of measuring required standoff from explosives is the use of “K-factors,” also known as scaled distances. You might be in a discussion and hear someone say, “We’ll apply K40 Inhabited Building Distance to that structure.” Or “Is that K18 Intraline Distance, or K9 Barricaded Intraline Distance?” But what on earth are these K-factors? This blog post will focus on how they are used and how they are calculated.
The Intent of K-Factors
Explosives Safety Quantity-Distance (ESQD) planning seeks to establish standoff distances for various categories of people and structures. Someone that is related to the explosives operations is allowed to be closer to the explosives than is someone unrelated (e.g., the public). Explosives operations and storage facilities are allowed to be subjected to higher hazards than are allowed for public gathering places or critical infrastructure.
The US DoD (Department of Defense) and other agencies have established acceptance thresholds for blast overpressure for various categories of exposed personnel and facilities. (It is important to note that K-factors only model blast overpressure. They do not address fragmentation hazard distances or thermal effects. We’ll cover those in a another post.) For example, in general, the public is required to be separated from detonable explosives by a distance that would ensure that pressure no greater than 1.2 psi reaches them. This threshold is true whether it is 10 lbs or 10,000 lbs of explosives. This required distance is known as Inhabited Building Distance (IBD) for overpressure. Naturally, IBD has to be a greater distance for 10,000 lbs to achieve the same level of protection. But how much greater?
K-factors are a mathematical method of quickly calculating required standoff distances for overpressure hazards. A K-factor of 40 (also referred to as K40) is associated with the IBD requirement of 1.2 psi of overpressure. The required standoff distance to achieve an arriving overpressure of 1.2 psi can be calculated using the equation below. This process assumes that the explosive is bare (unconfined) TNT or has been converted to equivalent TNT. The cube root of the explosives weight (lbs) is multiplied by a factor of 40 to obtain the required distance (ft).

But does it really work? This might sound like it’s too easy to be accurate. Aren’t explosives supposed to be harder to figure out? Well, kind of. There are some limitations. But the basic math works very well because geometry is amazing. It’s all about hemispheres….
The Math….
When a detonation of energetic material occurs, the solid state of the material is nearly instantly converted to a gas with a huge release of energy. This gas is forced out in all directions at extreme velocities. For general explosives safety planning purposes, detonations happen at ground level. All the gas and energy that would push downward is reflected upward. The result is an expanding hemisphere of energy. This is referred to in blast dynamics as a hemispherical surface burst.

It's the basic repeatable shape of the hemisphere that makes the math work for calculating expected overpressure at varying distances. Decades of explosives testing have confirmed the applicability of this method.
The equation for the volume of a hemisphere is shown below.
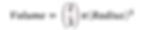
Rearranging this equation to solve for the radius, which in our case is the distance from the explosion, looks like this.

This is the same form as the K-factor equation. Okay, but what does all this mean?
The difference in required standoff between a small quantity of explosives and large quantity of explosives scales in the same manner as the volume of a hemisphere. That’s why these K-factors are often referred to as scaled distances.
Let’s see an example.
The following table shows varying explosives quantities (TNT equivalents) and the required K40 standoff at which the blast overpressure is expected to be approximately 1.2 psi. Notice that doubling the explosive weight does not double the required standoff distance. In fact, the required distance isn’t doubled unless you scale the quantity by just less than a full order of magnitude (actually a factor of 8 – based on the cube root). This is because when you double the amount of explosives, you are doubling the theoretical volume of the hemisphere at the point of 1.2 psi. But doubling the volume of a hemisphere does not double the radius (it only increases it by about 26%).

Applying K-Factors
With this ability to determine consistent standoff distances for any quantity of explosives, criteria can be developed. The US DoD has established the following overpressure requirements for varying exposure types.

The various DoD criteria manuals (e.g. DESR (Defense Explosives Safety Regulation) 6055.09) establish the rules for what types of facilities and personnel are allowed at various levels of blast overpressure. You can read another one of our blog posts to learn more about these various exposure levels. But the user of the criteria can use the specified K-factor to quickly determine the required standoff. The standoffs for various exposure types use the same cube root value of the explosive weight. The result is simply multiplied by the appropriate K-factor.

Other Standards
K-factors are extremely useful for calculating requirements associated with hemispherical effects like overpressure. But they do not represent other hazards such as fragmentation and thermal effects.
The universal nature of the math related to a hemisphere means that this principle is also used in many other explosives criteria standards around the world. The most common is the AASTP-1 (Allied Ammunition Storage and Transport Publication) criteria associated with NATO (North Atlantic Treaty Organization). AASTP-1 criteria are widely used and are based in metric units. The scaled distance factors are sometimes referred to as Q-factors due to the criteria referring to the explosive weight as the Quantity (Q) in the NATO equations.
However, the standoff distances for overpressure hazards do not match between the US DoD and the NATO AASTP-1 criteria. This is not because there is a difference in the math, but rather because AASTP-1 has different acceptable levels of overpressure for various exposures. For example, the equivalent IBD requires the public to be located farther from the explosive event, such that only approximately 0.9 psi reaches that point.
Contact The Schreifer Group
If you would like to learn more about these topics or how we can help with your site planning needs, contact The Schreifer Group. And check out our other articles on explosives safety topics. Please visit our website to learn more about how we can help. Our growing team of five explosives safety SMEs are ready to support you.